Effects of Driving Style on Energy Consumption and CO2 Emissions
DOI:
https://doi.org/10.17815/CD.2022.137Keywords:
energy consumption, CO2 emissions, velocity and acceleration distribution, traffic cellular automataAbstract
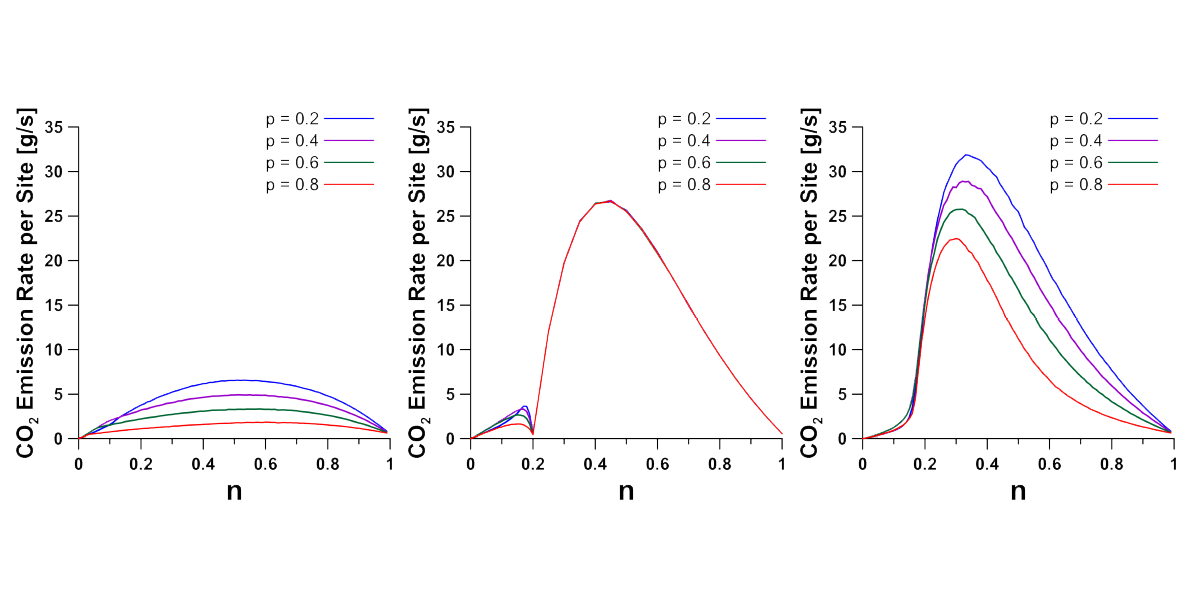
The tractive force developed by energy consumption (EC) in a car engine produces its acceleration and sustains the motion against velocity dependent resistance forces. In internal combustion engines, fuel burning entails pollutant emissions (PE) released into the atmosphere. In vehicular traffic, EC and PE depend on the driving style. This paper assumed that the transition rules in a traffic cellular automata (TCA) represent a driving style, and its effect on EC and PE in TCA is studied. Extending empirical relationships, we proposed models to estimate EC and PE in TCA from the velocity and acceleration distributions, which we obtained by computer simulations for three well-known TCA. The Nagel-Schreckenberg (NS) and Fukui-Ishibashi (FI) models, and a variant (NS+FI) defined by combining the NS and FI rules, were considered. The FI driving style revealed EC and CO2 emission rates dependent on the stochastic delay (p) only for low vehicular densities. We also detected that the larger EC and CO2 emission rates were 45.4 kW and 26.7 g/s with no dependence on p. With NS and NS+FI driving styles, the larger energy consumption and CO2 emission rates occurred for small stochastic delays, 18.4 kW and 6.6 g/s and 61.1kW and 30.2 g/s for p = 0.2. On average, for NS, FI, and NS+FI models (p = 0.2), we obtained energy consumptions of 1.88, 2.60, and 2.76 MJ/km, fuel consumptions of 0.08, 0.12, and 0.13 L/km, and CO2 emissions of 0.158, 0.460, and 0.562 kgCO2/km. Our results agree with those (3.37 MJ/km and 0.235 kgCO2/km) of petrol combustion car engines at 10 km/L. This work may help in designing flow and driving style scenarios to optimize vehicular traffic EC and reduce PE.
References
R.A. Alpher: Robert Herman. Physics Today 50, 77 (1997). doi:10.1063/1.881863.
I. Prigogine, R.C. Herman: Kinetic Theory of Vehicular Traffic. Elsevier (1971).
M. Cremer, J. Ludwig: A fast simulation model for traffic flow on the basis of boolean operations. Mathematics and Computers in Simulation 28, 297-303. doi:10.1016/0378-4754(86)90051-0.
K. Nagel, M. Schreckenberg: A cellular automaton model for freeway traffic. J. Phys. I. 2 2221–2229 (1992). doi:10.1051/jp1:1992277.
M. Fukui, Y. Ishibashi: Traffic Flow in 1D Cellular Automaton Model Including Cars Moving with High Speed. J. Phys. Soc. Japan. 65 1868–1870 (1996). doi:10.1143/JPSJ.65.1868.
D. Chowdhury, L. Santen, A. Schadschneider: Statistical physics of vehicular traffic and some related systems. Phys. Rep. 329 199–329 (2000). doi:10.1016/S0370-1573(99)00117-9.
D. Helbing: Traffic and related self-driven many-particle systems. Rev. Mod. Phys. 73 1067–1141 (2001). doi:10.1103/RevModPhys.73.1067.
T. Nagatani: The physics of traffic jams. Reports Prog. Phys. 65 1331–1386 (2002). doi:10.1088/0034-4885/65/9/203.
K. Nagel, P. Wagner, R. Woesler: Still Flowing: Approaches to Traffic Flow and Traffic Jam Modeling. Oper. Res. 51 681–710 (2003). doi:10.1287/opre.51.5.681.16755.
R. Barlovic, L. Santen, A. Schadschneider, M. Schreckenberg: Metastable states in cellular automata for traffic flow. Eur. Phys. J. B. 5 793–800 (1998). doi:10.1007/s100510050504.
O. Biham, A.A. Middleton, D. Levine: Self-organization and a dynamical transition in traffic-flow models. Phys. Rev. A. 46 R6124–R6127 (1992). doi:10.1103/PhysRevA.46.R6124.
B.-H. Wang, L. Wang, P.M. Hui: One-Dimensional Fukui-Ishibashi Traffic Flow Model. J. Phys. Soc. Japan. 66 3683–3684 (1997). doi:10.1143/JPSJ.66.3683.
R. Jiang, M.-B. Hu, H.M. Zhang, Z.-Y. Gao, B. Jia, Q.-S. Wu, B. Wang, M. Yang: Traffic Experiment Reveals the Nature of Car-Following. PLoS One. 9 e94351 (2014). doi:10.1371/journal.pone.0094351.
K. Ahn, H. Rakha, A. Trani, M. Van Aerde: Estimating Vehicle Fuel Consumption and Emissions based on Instantaneous Speed and Acceleration Levels. J. Transp. Eng. 128 182–190 (2002). doi:10.1061/(ASCE)0733-947X(2002)128:2(182).
A. Danczyk, D. Levinson, V. der Hoorn: Fundamentals of Transportation/Grade. WIKIBOOKS (2009). http://en.wikibooks.org/w/index.php?oldid=1436014.
H. Rakha, I. Lucic, S.H. Demarchi, J.R. Setti, M. Van Aerde: Vehicle Dynamics Model for Predicting Maximum Truck Acceleration Levels. J. Transp. Eng. 127 418–425 (2001). doi:10.1061/(ASCE)0733-947X(2001)127:5(418).
Effects of Driving Style on Energy Consumption and CO2 Emissions 31
K. Zhang, H.C. Frey: Road Grade Estimation for On-Road Vehicle Emissions Modeling Using Light Detection and Ranging Data. J. Air Waste Manage. Assoc. 56 777–788 (2006). doi:10.1080/10473289.2006.10464500.
K. Nagel: Particle hopping models and traffic flow theory. Phys. Rev. E. 53 4655–4672 (1996). doi:10.1103/PhysRevE.53.4655.
A. Salcido, E. Hernandez-Zapata, S. Carreon-Sierra: Exact results of 1D traffic cellular automata: The low-density behavior of the Fukui–Ishibashi model. Phys. A Stat. Mech. Its Appl. 494 276–287 (2018). doi:10.1016/j.physa.2017.11.162.
A. Schadschneider, M. Schreckenberg: Cellular automation models and traffic flow. J. Phys. A. Math. Gen. 26 L679–L683 (1993). doi:10.1088/0305-4470/26/15/011.
M. Schreckenberg, A. Schadschneider, K. Nagel, N. Ito: Discrete stochastic models for traffic flow. Phys. Rev. E. 51 2939–2949 (1995). doi:10.1103/PhysRevE.51.2939.
L.C.Q. Vilar, A.M.C. de Souza: Cellular automata models for general traffic conditions on a line. Phys. A Stat. Mech. Its Appl. 211 84–92 (1994). doi:10.1016/0378-4371(94)90069-8.
B.A. Toledo, E. Cerda, J. Rogan, V. Munoz, C. Tenreiro, R. Zarama, J.A. Valdivia: Phys. Rev. E 75 026108 (2007). doi:10.1103/PhysRevE.75.026108.
W. Shi, Y. Xue: Study on stability and energy consumption in typical car-following models. Physica A 381 (2007) 399–406. doi:10.1016/j.physa.2007.02.106.
W. Zhang, W. Zhang, X. Yang: Energy dissipation in the deterministic and nondeterministic Nagel–Schreckenberg models. Phys. A Stat. Mech. Its Appl. 387 4657–4664 (2008). doi:10.1016/j.physa.2008.04.004.
W.-X. Zhu. Analysis of CO2 emission in traffic flow and numerical tests. Physica A 392 4787–4792 (2013). doi:10.1016/j.physa.2013.06.024.
T.Q. Tang, J.G. Li, Y.P. Wang, G.Z. Yu: Vehicle’s fuel consumption of car- following models. Science China Technological Sciences 56 1307-1312 (2013). doi:10.1007/s11431-013-5182-9.
T.Q. Tang, J.G. Li, H.J. Huang, X.B. Yang: A car-following model with real- time road conditions and numerical tests. Measurement 48 63-76 (2014). doi:10.1016/j.measurement.2013.10.035.
Y. Xue, S.-J. Kang, W.-Z. Lu, H.-D. He: Energy dissipation of traffic flow at an on-ramp. Physica A 398 (2014) 172–178. doi:10.1016/j.physa.2013.12.032.
T.Q. Tang, J.G. Li, S.C. Yang, H.Y. Shang: Effects of on-ramp on the fuel consumption of the vehicles on the main road under car-following model. Physica A 419 293–300 (2015). doi:10.1016/j.physa.2014.10.051.
T.Q. Tang, Z.Y. Yi, Q.F. Lin: Effects of signal light on the fuel consumption and emissions under car-following model. Physica A 469 200–205 (2017) doi:10.1016/j.physa.2016.11.025.
Z. Jin, Z. Yang, H. Ge: Energy consumption investigation for a new car-following model considering driver’s memory and average speed of the vehicles. Physica A 506 1038–1049 (2018). doi:10.1016/j.physa.2018.05.034.
X. Ma, H. Ge, R. Cheng: Influences of acceleration with memory on stability of traffic flow and vehicle’s fuel consumption. Physica A 525 143–154 (2019). doi:10.1016/j.physa.2019.03.024.
Y. Xue, X. Wang, B. Cen, P. Zhang, H. He: Study on fuel consumption in the Kerner–Klenov–Wolf three-phase cellular automaton traffic flow model. Nonlinear Dyn. 102 393–402 (2020). doi:10.1007/s11071-020-05947-2.
Y. Qiao, Y. Xue, X. Wang, B. Cen, Y. Wang, W. Pan, Y. Zhang: Investigation of PM emissions in cellular automata model with slow-to-start effect. Phys. A Stat. Mech. Its Appl. 574 125996 (2021). doi:10.1016/j.physa.2021.125996.
M. Takayasu, H. Takayasu: 1/f Noise in a Traffic Model. Fractals 01 860–866 (1993). doi:10.1142/S0218348X93000885.
L. Int Panis, S. Broekx, R. Liu: Modelling instantaneous traffic emission and the influence of traffic speed limits. Sci. Total Environ. 371 270–285 (2006). doi:10.1016/j.scitotenv.2006.08.017.
N. Lakouari, O. Oubram, A. Bassam, S.E. Pomares Hernandez, R. Marzoug, H. Ez- Zahraouy: Modeling and simulation of CO2 emissions in roundabout intersection. J. Comput. Sci. 40 101072 (2020).doi:10.1016/j.jocs.2019.101072.
H. Binoua, H. Ez-Zahraouy, A. Khallouk, N. Lakouari: Carbon dioxide emission in a single-lane cellular automaton model with a series of traffic lights. Int. J. Mod. Phys. C. 31 2050154 (2020). doi:10.1142/S0129183120501545.
M.J. Klein: Principle of Detailed Balance. Phys. Rev. 97 1446–1447 (1955). doi:10.1103/PhysRev.97.1446.
N. Kidambi, R.L. Harne, Y. Fujii, G.M. Pietron, K.W. Wang: Methods in Vehicle Mass and Road Grade Estimation. SAE Int. J. Passeng. Cars - Mech. Syst. 7 2014- 01–0111 (2014). doi:10.4271/2014-01-0111.
US-EPA, Greenhouse Gas Inventory Guidance. Direct Emissions from Mobile Combustion Sources, US EPA Center for Corporate Climate Leadership (2016).
N. Eissfeldt, R. Schrader: Calculation of street traffic emissions with a queuing model. Comput. Technol. 7 5-15 (2002).
A. Salcido, S. Carreon-Sierra: Air Pollutant Emissions in the Fukui-Ishibashi and Nagel-Schreckenberg Traffic Cellular Automata. J. Appl. Math. Phys. 05 2140-2161 (2017). doi:10.4236/jamp.2017.511175.
A. Ilachinski: Cellular Automata. A Discrete Universe. WORLD SCIENTIFIC (2001). doi:10.1142/4702.
F. Bagnoli: Cellular Automata. In: Nonlinear Workbook. WORLD SCIENTIFIC. 257–274 (2011). doi:10.1142/9789814335799 0010.
M. Cremer, J. Ludwig: A fast simulation model for traffic flow on the basis of boolean operations. Math. Comput. Simul. 28 297–303 (1986). doi:10.1016/0378-4754(86)90051-0.
M. Sasvari, J. Kertesz: Cellular automata models of single-lane traffic. Phys. Rev. E. 56 4104–4110 (1997). doi:10.1103/PhysRevE.56.4104.
C.-J. Fu, B.-H. Wang, C.-Y. Yin, T. Zhou, B. Hu, K. Gao, P.M. Hui, C.- K. Hu: Analytical studies on a modified Nagel–Schreckenberg model with the Fukui–Ishibashi acceleration rule. Chaos, Solitons & Fractals. 31 772–776 (2007). doi:10.1016/j.chaos.2005.10.062.
N. Treiber, A. Kesting, C. Thiemann: How much does traffic congestion increase fuel consumption and emissions? Applying a fuel consumption model to the NGSIM trajectory data. In: Transportation Research Board 87th Annual Meeting, 17 (2008).
M. Nyhan, S. Sobolevsky, C. Kang, P. Robinson, A. Corti, M. Szell, D. Streets, Z. Lu, R. Britter, S.R.H. Barrett, C. Ratti: Predicting vehicular emissions in high spatial resolution using pervasively measured transportation data and microscopic emissions model. Atmos. Environ. 140 352–363 (2016). doi:10.1016/j.atmosenv.2016.06.018.
W. Pan, Y. Xue, H.-D. He, W.-Z. Lu: Impacts of traffic congestion on fuel rate, dissipation and particle emission in a single lane based on Nasch Model. Phys. A Stat. Mech. Its Appl. 503 154–162 (2018). doi:10.1016/j.physa.2018.02.199.
V. Astarita, V.P. Giofre`, G. Guido, A. Vitale: A Single Intersection Cooperative- Competitive Paradigm in Real Time Traffic Signal Settings Based on Floating Car Data. Energies. 12 409 (2019). doi:10.3390/en12030409.
A. Madani, N. Moussa: Simulation of fuel consumption and engine pollutant in cellular automaton. J. Theor. Appl. Inf. Technol. 35 250–257 (2012).
X. Wang, Y. Xue, B. Cen, P. Zhang, H. He: Study on pollutant emissions of mixed traffic flow in cellular automaton. Phys. A Stat. Mech. Its Appl. 537 122686 (2020). doi:10.1016/j.physa.2019.122686.
D. Chu, Z. Deng, Y. He, C. Wu, C. Sun, Z. Lu: Curve speed model for driver assistance based on driving style classification. IET Intell. Trans. Syst. 11, 501–510 (2017). doi:10.1049/iet-its.2016.0294
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2022 Susana Carreón-Sierra, Alejandro Salcido

This work is licensed under a Creative Commons Attribution 4.0 International License.
Authors contributing to Collective Dynamics agree to publish their articles under the Creative Commons Attribution 4.0 license.
This license allows:
Share — copy and redistribute the material in any medium or format
Adapt — remix, transform, and build upon the material
for any purpose, even commercially.
The licensor cannot revoke these freedoms as long as you follow the license terms.
Authors retain copyright of their work. They are permitted and encouraged to post items submitted to Collective Dynamics on personal or institutional websites and repositories, prior to and after publication (while providing the bibliographic details of that publication).








