Coordination Game in Bidirectional Flow
DOI:
https://doi.org/10.17815/CD.2016.8Keywords:
evolutionary game dynamics, cellular automata, bidirectional flow, self-driven particlesAbstract
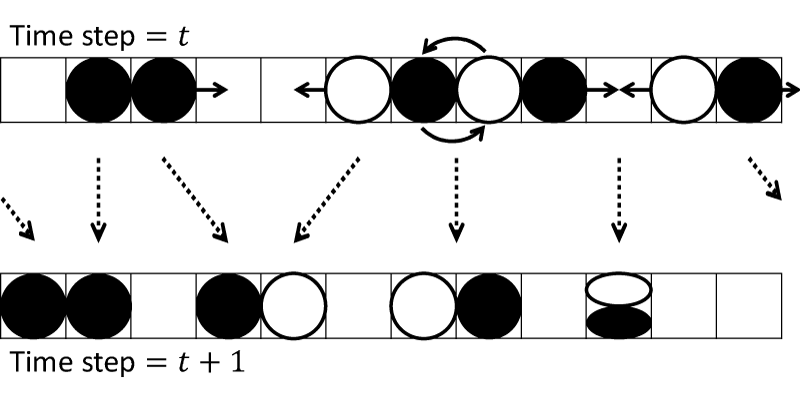
We have introduced evolutionary game dynamics to a one-dimensional cellular- automaton to investigate evolution and maintenance of cooperative avoiding behavior of self-driven particles in bidirectional flow. In our model, there are two kinds of particles, which are right-going particles and left-going particles. They often face opponent particles, so that they swerve to the right or left stochastically in order to avoid conflicts. The particles reinforce their preferences of the swerving direction after their successful avoidance. The preference is also weakened by memory-loss effect.
Result of our simulation indicates that cooperative avoiding behavior is achieved, i.e., swerving directions of the particles are unified, when the density of particles is close to 1/2 and the memory-loss rate is small. Furthermore, when the right-going particles occupy the majority of the system, we observe that their flow increases when the number of left-going particles, which prevent the smooth movement of right-going particles, becomes large. It is also investigated that the critical memory-loss rate of the cooperative avoiding behavior strongly depends on the size of the system. Small system can prolong the cooperative avoiding behavior in wider range of memory-loss rate than large system.
References
Mattli, W., Büthe, T.: Setting International Standards: Technological Rationality or Primacy of Power? World Polit. 56(1), 1-42 (2003). doi:10.1353/wp.2004.0006
Bloomquist, K.M.: Tax Compliance as an Evolutionary Coordination Game: An Agent-Based Approach. Public Financ. Rev. 39(1), 25-49 (2011). doi:10.1177/1091142110381640
Bian, Y.T., Xu, L., Li, J.S.: Evolving dynamics of trading behavior based on coordination game in complex networks. Phys. A Stat. Mech. its Appl. 449, 281-290 (2016). doi:10.1016/j.physa.2015.12.113
Helbing, D.: Traffic and related self-driven many-particle systems. Rev. Mod. Phys. 73(4), 1067-1141 (2001). doi:10.1103/RevModPhys.73.1067
Seyfried, A., Steffen, B., Klingsch, W., Boltes, M.: The fundamental diagram of pedestrian movement revisited. J. Stat. Mech. Theory Exp. 2005(10), P10002-P10002 (2005). doi:10.1088/1742-5468/2005/10/P10002
Antonini, G., Bierlaire, M., Weber, M.: Discrete choice models of pedestrian walking behavior. Transp. Res. Part B Methodol. 40(8), 667-687 (2006). doi:10.1016/j.trb.2005.09.006
Yanagisawa, D., Kimura, A., Tomoeda, A., Nishi, R., Suma, Y., Ohtsuka, K., Nishinari, K.: Introduction of frictional and turning function for pedestrian outflow with an obstacle. Phys. Rev. E 80(3), 036110 (2009). doi:10.1103/PhysRevE.80.036110
Schadschneider, A., Chowdhury, D., Nishinari, K., Santen, L.: Stochastic Transport in Complex Systems. Elsevier, Amsterdam/Oxford (2010)
Helbing, D., Molnár, P.: Social force model for pedestrian dynamics. Phys. Rev. E 51(5), 4282-4286 (1995). doi:10.1103/PhysRevE.51.4282
Blue, V.J., Adler, J.L.: Cellular automata microsimulation for modeling bi-directional pedestrian walkways. Transp. Res. Part B Methodol. 35(3), 293-312 (2001). doi:10.1016/S0191-2615(99)00052-1
Flötteröd, G., Lämmel, G.: Bidirectional pedestrian fundamental diagram. Transp. Res. Part B Methodol. 71(2015), 194-212 (2015). doi:10.1016/j.trb.2014.11.001
Morton, N.A., Hendy, S.C.: Symmetry Breaking in Pedestrian Dynamics (2016). URL http://arxiv.org/abs/1605.05437
Hoogendoorn, S., Daamen, W.: Self-Organization in Pedestrian Flow. In: Traffic Granul. Flow '03, pp. 373-382. Springer-Verlag Berlin Heidelberg (2005). doi:10.1007/3-540-28091-X_36
Zhang, J., Seyfried, A.: Empirical Characteristics of Different Types of Pedestrian Streams. Procedia Eng. 62, 655-662 (2013). doi:10.1016/j.proeng.2013.08.111
Feliciani, C., Nishinari, K.: Phenomenological description of deadlock formation in pedestrian bidirectional flow based on empirical observation. J. Stat. Mech. Theory Exp. 2015(10), P10003 (2015). doi:10.1088/1742-5468/2015/10/P10003
Konno, T.: Coordination Always Occurs in a Two-Strategy Pure-Coordination Logit Game on Scale-Free Networks. Theor. Econ. Lett. 05(04), 561-570 (2015). doi:10.4236/tel.2015.54066
Hausman, J., McFadden, D.: Specification Tests for the Multinomial Logit Model. Econometrica 52(5), 1219 (1984). doi:10.2307/1910997
Schadschneider, A., Schreckenberg, M.: Cellular automation models and traffic flow. J. Phys. A. Math. Gen. 26(15), L679-L683 (1993). doi:10.1088/0305-4470/26/15/011
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2016 Daichi Yanagisawa

This work is licensed under a Creative Commons Attribution 4.0 International License.
Authors contributing to Collective Dynamics agree to publish their articles under the Creative Commons Attribution 4.0 license.
This license allows:
Share — copy and redistribute the material in any medium or format
Adapt — remix, transform, and build upon the material
for any purpose, even commercially.
The licensor cannot revoke these freedoms as long as you follow the license terms.
Authors retain copyright of their work. They are permitted and encouraged to post items submitted to Collective Dynamics on personal or institutional websites and repositories, prior to and after publication (while providing the bibliographic details of that publication).








